Explaining away

One morning you discover your lawn is wet. Maybe your up-hill neighbor drained his kiddie-pool. Or maybe it rained in the night. How do we find the truth about what happened? And what does this tell us about the greatest mysteries of the Cosmos?

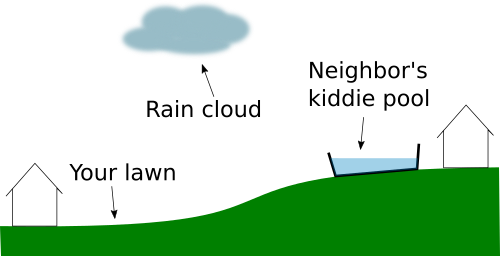
Perhaps yesterday's weather forecast said there would be a 20% chance of rain during the night. And since your neighbor is usually considerate, you estimate that there was a 5% chance that he would have drained his pool without notifying you in advance. And just to keep this simple, let's suppose there are no other possibilities. So, rain is four times more likely to be the right explanation, right?

Sure, but there's a lot more we can learn if we take the time to do a little math. So don't be lazy! Making yourself think formally is good for your brain. Let's do this the right way!

First, let's compute the a priori probability that your lawn might be wet. It turns out that it is possible for both events to simultaneously occur. (Maybe your neighbor drained his pool and it rained.) But if the grass were dry then we would know that neither event had occurred. So, we can use De Morgan's law to compute the probability that the grass would stay dry, and subtract from 1:

         p(wet) = 1 - (1 - p(pool)) * (1 - p(rain))

                = 1 - (1 - 0.05) * (1 - 0.2)

                = 0.24

Next, we can invoke Bayes' law to compute how likely each cause is to be responsible for your lawn being wet:

    p(pool|wet) = p(wet|pool) * p(pool) / p(wet)

                = 1 * 0.05 / 0.24

                = 0.2083

There is a 20.83% chance that your neighbor drained his pool.

    p(rain|wet) = p(wet|rain) * p(rain) / p(wet)

                = 1 * 0.2 / 0.24

                = 0.83

And there is an 83.3% chance that it rained.

Why do these numbers sum to more than 1?
(0.2083 + 0.83 = 1.0416.) Because there is also a chance that they both occurred. It's not a big chance, but it is still a possibility.

Now, if skilled meteorologists predicted only a 20% chance of rain, how did you become 83.3% confident that it rained? Because you have additional information they didn't have. You know that your grass is wet. Something must explain that phenomenon. Sticking with only 20% confidence while standing on wet grass would simply not be rational.

And here we make the most important observation of this article: Yes, it is possible to quantify certainty and uncertainty with math! Bayesian statistics is turning epistemology from a branch of philosophy into a branch of science. No longer need we lean on philosophers to give us conflicting messages about how to know what is true--we can just do the $#@!'in math!

But just as you start thinking that you know what probably happened, suppose your neighbor walks by and says,

"Howdy, neighbor! Oh dear, it looks like I got your lawn all wet when I drained my pool last night. Sorry about that."

(Also, your neighbor is Ned Flanders. I forgot to mention that.) This new information changes things quite substantially! Of course, new information does not change whether it rained last night, but it most certainly does change what you know. So, let's adjust the math to accommodate this new information. (And this is where we start to see the philosophical implications of doing the math.)

Assuming you believe your neighbor, the wetness of your grass has now been explained. So your wet grass no longer tells you anything about whether or not it rained. (Rewind the math.) Now the only information you have to determine whether or not it rained is that weather forecast you remembered. (It said there was a 20% chance of rain.) So, the new more informed probability that it rained last night is 20%. When your neighbor told you he drained his pool, that changed your beliefs about whether it rained from 83.3% (meaning you mostly believe it rained) to 20% (meaning you mostly disbelieve it rained).

Let's repeat that with some emphasis: When your neighbor said he drained his pool, that changed your beliefs about whether it rained from 83.3% to 20%.

Does the weather care about your neighbor draining his pool?

No.

Is the weather aware that your neighbor drained his pool?

Obviously not.

Does your neighbor have some kind of power to influence the weather by draining his pool?

Nope.

And nothing about hearing what your neighbor did to his pool has any effect on the weather whatsoever.

So how in Bayes' holy name could information completely unrelated to the weather possibly change what you believed about the weather? How did the previously most-likely explanation, rain, suddenly become an unlikely explanation, when you didn't even investigate the weather?

...hmm?

Because your neighbor explained away the rain hypothesis. When you understood how your grass became wet, the rain explanation no longer served a useful purpose. And the math backs up this intuition. This is the right way to reason. Any other way to reason about it would deviate from reality.

Implications

Some people have the mistaken idea that science cannot say anything about God because God is a supernatural being, and science only investigates natural phenomena.

This sounds like good reasoning, doesn't it?

It's not.

Why is it rational to believe in God? Because, we exist. (This is like the wet grass.) We live on a planet that is covered with life. We are conscious, and our hearts yearn for meaning. And there is something that seems extraordinary about our feelings, our experiences, and our sense of morality. There must be an extraordinary explanation for all these extraordinary observed phenomena! These things demand explanations. And God is an explanation. These observations make it rational to believe in God.

Considering so many extraordinary observations, the likelihood that God exists is called his a posteriori (pronounced like "apple posterior story") likelihood. But what if there was no supporting evidence? What if the only reason to believe in God was the self-evident degree to which a God probably exists anyway. This is called his a priori (pronounced like "apple preview story") likelihood.

As science increasingly finds natural explanations for the phenomena that made it rational to believe in God, the rationality of belief in God shifts from the a posteriori back toward the a priori likelihood. (This is like the neighbor revealing what really made the grass wet.)

But isn't it possible that both factors co-occurred? For example, couldn't God be the cause of evolution?

Yes, that is a possibility to consider too. But unless there is some reason to suppose otherwise, the probability of having a superfluous cause will always be smaller than the probability of not having that superfluous cause. This is why one of the most effective ways to maintain faith in God is to deliberately avoid learning too much about the compelling explanations that science has for natural phenomena. But hiding from science is really more of an expression of fear than of faith, isn't it? What do we see if we exercise the faith to let the evidence speak for itself?

We see that cosmologists have developed models showing that the Earth probably accreted naturally like the distant solar systems we can observe. We see that Geologists confirm that the age of the Earth is consistent with this explanation. Evolutionary biologists have evidence that all life on this planet evolved from common origins. Neuroscientists have started to find evidence that our brains are responsible for consciousness, casting doubt on the role of spirits. Anthropologists have evidence of populations that lived through Noah's flood. Phylogeneticists have managed to sequence the genome, and have found strong statistical evidence that was predicted by the theory of evolution long before we even had that capability. psychologists have discovered that all humans are vulnerable to confirmation bias, which explains how people become so certain that they have the truth, even though they all believe in different religions. Computer scientists have managed to simulate evolution and discover that genetic algorithms are more effective at optimizing large numbers of variables than the most intelligent human designers. Artificial intelligence has begun to achieve a great many of the cognitive capabilities that humans exhibit in functional machines. And every other branch of science contributes something that suggests things in this world happen naturally. ...every one of them.

Which of these branches of science directly investigates supernatural matters? None of them. And yet, every one of them explains away some of the reasons people suppose there might be a God.

Just to be absolutely clear, none of the discoveries in all of science change the probability that God is really there. But all of them change what we know. (In fact that's their job.) As science approaches finding natural explanations for all our observations, the rationality of believing in God shifts from its a posteriori likelihood back to the a priori likelihood. That is, it approaches exactly what it would be if there was no Earth, no life, and no other reason whatsoever to suppose that the God explanation was needed.

...and that's pretty flimsy.

It is true that science does not investigate the supernatural. But that does not mean the God hypothesis is safe from science. Every natural explanation we find explains away more of the reasons people believe in God. Science has not yet explained away every reason, but there is no branch of science that completely leaves him alone. And the current trajectory does not look very good for the God hypothesis.

Some technical notes

1. Conditional independence: When we did the math, we assumed the pool does not influence the rain, and the rain does not cause the pool to be drained. Explaining away is a valid principle even when there is no conditional independence, but the math is a little more hairy. I wanted to keep this example as simple as possible. But if you want to learn more about Bayesian inference, it's a great topic to study.
2. Laws vs approximations: DeMorgan's law, Bayes' law, and the arithmetic operations we used are laws, not approximations. This means that if we made no math errors, our conclusions contained no uncertainty beyond that which was already present in our assumptions. In other words, this is not an estimation technique. It is a method for computing what your probabilistic assumptions actually imply.
3. Two-edged sword: Explaining away can work in both directions. (In my wet grass example, the neighbor's pool explanation, which was my analog for intelligent intervention, actually explained away the rain explanation, which was my analog for natural phenomenon.) That's why people who focus on science tend to depart from religious explanations, and people who focus on religion tend to depart from scientific explanations. But whichever way you go, it is clear that religion and science are not compatible without resorting to mental gymnastics of ever-growing complexity.
4. Where do we get numbers that express what pieces of evidence say about the existence of God? Bayesian inference shows how to correctly compute the implications of your assumptions, without introducing any bias that was not already present in your assumptions. It does not tell you what assumptions you should make. So the burden still falls on you to decide which evidence to consider, and how strongly to weight it. If you want to adjust the weights of your evidence until you get the results you prefer, that's a problem of intellectual integrity, not a problem with Bayesian inference. And even if you cannot come up with meaningful probabilities, Bayesian inference still shows us how to reason in the face of uncertainty. One thing it clearly demonstrates is that beliefs about supernatural phenomena are not immune to the investigations of science, even if science only investigates natural phenomena. And that was the main point I was making in this article.
5. Why is p(wet|rain) = 1? When I applied Bayes' law, I assumed that rain always makes the grass wet. If there is some chance that the grass might dry out before you investigate, this term could be adjusted to reflect that. Also, if there were some chance that your neighbor might drain his pool, but route the water around your lawn, you could set p(wet|pool) to some value smaller than 1, and the same principle would still work. In the real world, almost no factor of cause-and-effect is absolutely certain. This is precisely why the principle of explaining away is so critical to understand when we try to reason about what is true in a Universe full of uncertainty.